Minimal Universal Exponent

Source

Link

This was a project for a number theory course: MATH 4150 at Georgia Tech. The goal was to compute the minimal universal exponent for a number, also known as the Carmichael function.

The minimal universal exponent of a positive integer $n$ is the smallest positive integer $m$ such that:

This Carmichael function, we'll refer to it as $\lambda(n) = m$, is instrumental to the generation of RSA keys.

In order to compute the function, we have to break down $n$ to it's prime factors. Then by the Chinese Remainder Theorem, we can compute the minimal universal exponent using the least common multiple of the prime factors' exponents.

Then for any prime factor $\lambda(p_i^{r_i})$ corresponds with Euler's totient for that prime factor.

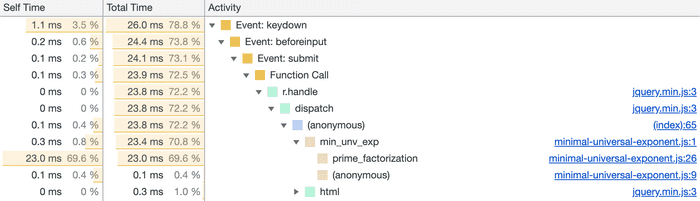
This means that the majority of the work by far is in factoring $n$ since computing the lcm and Euler's totient for the prime factors is trivial. This is evident in the Chrome DevTools profile:

For reference, here is the function for computing the minimal universal exponent:

function min_unv_exp(num) {
  /* Returns a number representing the minimal universal exponent
   * of a positive integer num */

  var e = 1; /* This will be the returned exponent, note: needs to be
    one for the first lcm to work */

  /* Iterate through prime factors, computing lcm of each totient */
  prime_factorization(num).forEach(function (factor) {
    if (factor.p === 2) {
      if (factor.e == 2) {
        e = lcm(e, 2);
      } else if (factor.e > 2) {
        e = lcm(e, Math.pow(2, factor.e - 2));
      }
    } else {
      e = lcm(e, Math.pow(factor.p, factor.e - 1) * (factor.p - 1));
    }
  });

  return e;
}

Digging into the prime factorization function the fastest way I could implement it was using a accumulator approach:

function prime_factorization(n) {
  /* Returns an array of prime factors (in least sorted order)
   * where each factor is an object of the form
   * {'p': 7, 'e': 4}
   * where p is the prime base and e is the exponent for p in the factorization
   */
  var factors = [];
  for (var p = 2; p <= n; p++) {
    var e = 0;
    while (n % p == 0) {
      n = n / p;
      e++;
    }
    if (e > 0) {
      factors.push({ p: p, e: e });
    }
  }
  return factors;
}

This relies on the observation that dividing $n$ by its the smallest prime factor $p_1^{r_1}$ the smallest number greater than $p_1$ that divides $n/p_1^{r_1}$ is necessarily a prime factor of $n$.

A quick proof for this follows from contradiction. Let $p_1^{r_1}$ be the factor of $n$ such that $p_1$ is minimal and $r_1$ is maximal.

Now assume $q > p_1$ is the next smallest divisor of $n$ and $q$ is not prime.

Since $q$ is composite, there must exist a prime factor $\phi < q$.

Since $\phi|n \,\rarr\, \phi > p_1$ otherwise $p_1$ would not be the smallest prime factor of n.

However, since $q > \phi > p_1$ and $\phi|n$ that means $q$ is not the next smallest divisor of $n$ unless $q=\phi$ in which case $q$ is prime breaking our assumption that $q$ is composite. ∎